This article is about the electronic component. For the physical phenomenon, see capacitance. For an overview of various kinds of capacitors, see types of capacitor.
| Capacitor | |
|---|---|
 Modern capacitors, by a cm rule | |
| Type | Passive |
| Invented | Ewald Georg von Kleist (October 1745) |
| Electronic symbol | |
A capacitor (formerly known as condenser) is a device for storing electric charge. The forms of practical capacitors vary widely, but all contain at least two conductors separated by a non-conductor. Capacitors used as parts of electrical systems, for example, consist of metal foils separated by a layer of insulating film.
A capacitor is a passive electronic component consisting of a pair of conductors separated by a dielectric (insulator). When there is a potential difference (voltage) across the conductors, a static electric field develops across the dielectric, causing positive charge to collect on one plate and negative charge on the other plate. Energy is stored in the electrostatic field. An ideal capacitor is characterized by a single constant value,capacitance, measured in farads. This is the ratio of the electric charge on each conductor to the potential difference between them.
Capacitors are widely used in electronic circuits for blocking direct current while allowingalternating current to pass, in filter networks, for smoothing the output of power supplies, in the resonant circuits that tune radios to particular frequencies and for many other purposes.
The capacitance is greatest when there is a narrow separation between large areas of conductor, hence capacitor conductors are often called "plates," referring to an early means of construction. In practice the dielectric between the plates passes a small amount ofleakage current and also has an electric field strength limit, resulting in a breakdown voltage, while the conductors and leads introduce an undesired inductance and resistance.
In October 1745, Ewald Georg von Kleist of Pomerania in Germany found that charge could be stored by connecting a high voltage electrostatic generator by a wire to a volume of water in a hand-held glass jar. Von Kleist's hand and the water acted as conductors and the jar as a dielectric (although details of the mechanism were incorrectly identified at the time). Von Kleist found, after removing the generator, that touching the wire resulted in a painful spark. In a letter describing the experiment, he said "I would not take a second shock for the kingdom of France."The following year, the Dutch physicist Pieter van Musschenbroek invented a similar capacitor, which was named the Leyden jar, after the University of Leiden where he worked.
Daniel Gralath was the first to combine several jars in parallel into a "battery" to increase the charge storage capacity. Benjamin Franklin investigated the Leyden jar and "proved" that the charge was stored on the glass, not in the water as others had assumed. He also adopted the term "battery", (denoting the increasing of power with a row of similar units as in a battery of cannon), subsequently applied to clusters of electrochemical cells.[ Leyden jars were later made by coating the inside and outside of jars with metal foil, leaving a space at the mouth to prevent arcing between the foils. The earliest unit of capacitance was the 'jar', equivalent to about 1 nanofarad.
Leyden jars or more powerful devices employing flat glass plates alternating with foil conductors were used exclusively up until about 1900, when the invention of wireless (radio) created a demand for standard capacitors, and the steady move to higher frequencies required capacitors with lower inductance. A more compact construction began to be used of a flexible dielectric sheet such as oiled paper sandwiched between sheets of metal foil, rolled or folded into a small package.
Early capacitors were also known as condensers, a term that is still occasionally used today. The term was first used for this purpose byAlessandro Volta in 1782, with reference to the device's ability to store a higher density of electric charge than a normal isolated conductor.
[Theory of operation
Main article: Capacitance
A capacitor consists of two conductors separated by a non-conductive region. The non-conductive region is called the dielectric or sometimes the dielectric medium. In simpler terms, the dielectric is just an electrical insulator. Examples of dielectric mediums are glass, air, paper, vacuum, and even a semiconductor depletion region chemically identical to the conductors. A capacitor is assumed to be self-contained and isolated, with no net electric charge and no influence from any external electric field. The conductors thus hold equal and opposite charges on their facing surfaces,and the dielectric develops an electric field. In SI units, a capacitance of one farad means that one coulomb of charge on each conductor causes a voltage of one volt across the device.
The capacitor is a reasonably general model for electric fields within electric circuits. An ideal capacitor is wholly characterized by a constant capacitance C, defined as the ratio of charge ±Q on each conductor to the voltage V between them:
Sometimes charge build-up affects the capacitor mechanically, causing its capacitance to vary. In this case, capacitance is defined in terms of incremental changes:
[edit]Energy storage
Work must be done by an external influence to "move" charge between the conductors in a capacitor. When the external influence is removed the charge separation persists in the electric field and energy is stored to be released when the charge is allowed to return to itsequilibrium position. The work done in establishing the electric field, and hence the amount of energy stored, is given by:
]Current-voltage relation
The current i(t) through any component in an electric circuit is defined as the rate of flow of a charge q(t) passing through it, but actual charges, electrons, cannot pass through the dielectric layer of a capacitor, rather an electron accumulates on the negative plate for each one that leaves the positive plate, resulting in an electron depletion and consequent positive charge on one electrode that is equal and opposite to the accumulated negative charge on the other. Thus the charge on the electrodes is equal to the integral of the current as well as proportional to the voltage as discussed above. As with any antiderivative, a constant of integration is added to represent the initial voltage v (t0). This is the integral form of the capacitor equation,
 .
.
Taking the derivative of this, and multiplying by C, yields the derivative form,]
 .
.
The dual of the capacitor is the inductor, which stores energy in the magnetic field rather than the electric field. Its current-voltage relation is obtained by exchanging current and voltage in the capacitor equations and replacing C with the inductance L.
DC circuits
See also: RC circuit
A series circuit containing only a resistor, a capacitor, a switch and a constant DC source of voltage V0 is known as a charging circuit. If the capacitor is initially uncharged while the switch is open, and the switch is closed at t = 0, it follows from Kirchhoff's voltage law that
Taking the derivative and multiplying by C, gives a first-order differential equation,
At t = 0, the voltage across the capacitor is zero and the voltage across the resistor is V0. The initial current is then i (0) =V0 /R. With this assumption, the differential equation yields
where τ0 = RC is the time constant of the system.
As the capacitor reaches equilibrium with the source voltage, the voltage across the resistor and the current through the entire circuit decay exponentially. The case of discharging a charged capacitor likewise demonstrates exponential decay, but with the initial capacitor voltage replacing V0 and the final voltage being zero.
AC circuits
See also: reactance (electronics) and electrical impedance#Deriving the device specific impedances
Impedance, the vector sum of reactance and resistance, describes the phase difference and the ratio of amplitudes between sinusoidally varying voltage and sinusoidally varying current at a given frequency. Fourier analysis allows any signal to be constructed from a spectrum of frequencies, whence the circuit's reaction to the various frequencies may be found. The reactance and impedance of a capacitor are respectively
where j is the imaginary unit and ω is the angular velocity of the sinusoidal signal. The - j phase indicates that the AC voltage V = Z I lags the AC current by 90°: the positive current phase corresponds to increasing voltage as the capacitor charges; zero current corresponds to instantaneous constant voltage, etc.
Note that impedance decreases with increasing capacitance and increasing frequency. This implies that a higher-frequency signal or a larger capacitor results in a lower voltage amplitude per current amplitude—an AC "short circuit" or AC coupling. Conversely, for very low frequencies, the reactance will be high, so that a capacitor is nearly an open circuit in AC analysis—those frequencies have been "filtered out".
Capacitors are different from resistors and inductors in that the impedance is inversely proportional to the defining characteristic, i.e.capacitance.
[edit]Parallel plate model
The simplest capacitor consists of two parallel conductive plates separated by a dielectric withpermittivity ε (such as air). The model may also be used to make qualitative predictions for other device geometries. The plates are considered to extend uniformly over an area A and a charge density ±ρ = ±Q/A exists on their surface. Assuming that the width of the plates is much greater than their separation d, the electric field near the centre of the device will be uniform with the magnitude E = ρ/ε. The voltage is defined as the line integral of the electric field between the plates
Solving this for C = Q/V reveals that capacitance increases with area and decreases with separation
 .
.
The capacitance is therefore greatest in devices made from materials with a high permittivity, large plate area, and small distance between plates. However solving for maximum energy storage using Vd as the dielectric strength per distance
we see that the maximum energy is a function of dielectric volume, permittivity, and dielectric strength per distance. So increasing the plate area while decreasing the separation between the plates while maintaining the same volume has no change on the amount of energy the capacitor can store. Care must be taken when increasing the plate separation so that the above assumption of the distance between plates being much smaller than the area of the plates is still valid for these equations to be accurate.
NETWORKS
See also: Series and parallel circuits
- For capacitors in parallel
- Capacitors in a parallel configuration each have the same applied voltage. Their capacitances add up. Charge is apportioned among them by size. Using the schematic diagram to visualize parallel plates, it is apparent that each capacitor contributes to the total surface area.
- For capacitors in series
- Connected in series, the schematic diagram reveals that the separation distance, not the plate area, adds up. The capacitors each store instantaneous charge build-up equal to that of every other capacitor in the series. The total voltage difference from end to end is apportioned to each capacitor according to the inverse of its capacitance. The entire series acts as a capacitor smaller than any of its components.

- Capacitors are combined in series to achieve a higher working voltage, for example for smoothing a high voltage power supply. The voltage ratings, which are based on plate separation, add up. In such an application, several series connections may in turn be connected in parallel, forming a matrix. The goal is to maximize the energy storage utility of each capacitor without overloading it.








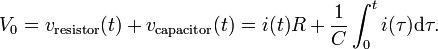

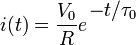


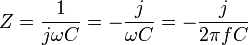

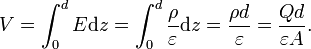
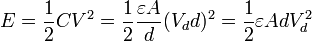



No comments:
Post a Comment